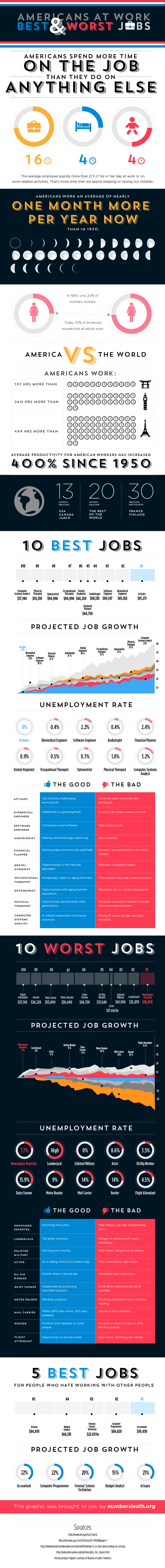
On the television game show Let’s Make a Deal, Monty Hall, the show’s best known host, used to present contestants with the following situation: the contestant would be presented with three doors behind one of which was a big prize (say a brand new car). Behind the other two doors was a small prize (say $100 in cash). The contestant got to choose one of these doors. If the contestant chose the correct door (i.e., the one with the big prize), she could keep it.
But to make things interesting, after the contestant chose a door, Monty Hall would open one of the other two doors, making sure that it was one with a small prize ($100 in cash). Hall could always find such a door because only one had a big prize and the other two had small prizes—so there would always be such a door regardless of whether the contestant chose the correct door.
Now, after Hall opened a door with a small prize, he gave the contestant the following option: she could stay with her initial choice of doors or switch to the other unopened door. Once she decided whether to stay or switch, all doors would be opened and the contestant would only receive the big prize if it was behind the door she had finally settled on. So the Monty Hall problem is this: should she stay with her original door or switch?
There’s been a huge amount written on this problem and many smart people, when initially presented with it, think it doesn’t matter whether the contestant stays with her initial door or switches. Regardless of the contestant’s choice of doors, they reason, Hall knows what’s behind all three doors and can always open one with a small prize behind it. People seem psychologically programmed to think it doesn’t matter whether to stay or switch doors.
And yet, it turns out that switching rather than staying with one’s initial door is a much more effective strategy for winning the big prize. Indeed, by switching you increase your chances of winning the big prize from 1/3 to 2/3. Why is this?
Think of it this way: Initially you know nothing about which door has the big prize behind it. You’re probability of getting the big prize is therefore 1/3. Moreover, if your strategy is to stay with your initial choice, your probability doesn’t change—in that case, it doesn’t matter whether Hall subsequently opens one of the doors.
But now think of what happens when you switch. Initially you had a 1/3 probability of choosing the right door. If you did happen to choose the right door initially, now switching loses you the big prize. So you now have a probability of 1/3 of not getting the big prize. But that means your probability of getting the big prize, by switching, is now 2/3. It’s gone up!
In a sense, with the switching strategy, you hope that your initial choice is wrong so that by switching you get it right. But initially, your chance of getting it right is only 1/3 and getting it wrong is 2/3. By switching, your chance of success is the same as getting it initially wrong, so your probability of getting it right has gone up to 2/3. Hence, if you ever appear as a contestant on Let’s Make a Deal, choose the switching strategy.
Of course, it’s unlikely that you’ll ever appear on Let’s Make a Deal. So what’s the take-home lesson from the Monty Hall problem that you can apply to your life? It’s this: whenever there’s some event that’s important to you and you get new information about it, this new information can change the event’s probability or perhaps even prompt a course of action on your part that changes the event’s probability.
If it’s an event you want to avoid, then you’ll want to bring the probability down. If it’s an event you want to have happen (as in winning the big prize), then you’ll want to bring the probability up. In any case, you’ll want to do the probability calculation and see precisely how the probability has changed in light of the new information.
The Monty Hall problem is counterintuitive in that people’s first reaction tends to be that it doesn’t really matter whether to switch or stay with one’s initial choice. In these situations, it’s therefore not enough trust one’s intuitions. One needs, rather, to get out paper and pencil and do the calculation.
Even extensive training in statistics tends not to hone our intuitions in situations like the Monty Hall problem. Indeed, trained statisticians do no better than the rest of us until they apply their training and calculate the precise probabilities in question. The Monty Hall problem thus shows how our intuitions can mislead us and how mathematics can correct those intuitions.
—–
References:
Edward J. Barbeau, Mathematical Fallacies, Flaws and Flimflam (Washington, DC: The Mathematical Association of America, 2000), 86–90.
Amos Tversky and Daniel Kahneman, “Judgment under uncertainty: Heuristics and biases,” Science 185 (1974): 1124–1131.